Here are some illustrations by myself and others, dealing with the Fibonacci sequence and the Golden Number, Phi.
A few months ago, I noticed the exponents of Phi encode the Fibonacci sequence.
The sequence gets closer to the golden number the larger the numbers get. This will work with any two starting numbers, like say 91 and 432, for example. You’ll find the same ratio emerge if you keep adding them together. (91+432 = 523, then, 432+523=955, etc) Already we are at 1.8 to 1. (955/523) A handful more steps and we’ll have Phi to more than 99.999% accuracy.
𝄞 If you had a million phalanges, your hand would look like King Midas.
Jain108 made a post on how, if you start with 2 and 10, you arrive at the actual numbers found in the exponents of Phi. Remember exponents are simply the number multiplied by itself n number of times. Phi cubed, or Phi to the third power, is just Phi x Phi x Phi. (1.618 x 1.618 x 1.618 = 4.236) See that was easy, now stop complaining how you’re bad at math.
Phi to the negative power is the same as saying, one divided by the Phi cubed, or whatever.

^Here’s some different ways to say the same thing. Notice how all equations only use Fibonacci numbers.

This does an excellent job of explaining where all these freaking numbers come from. (img Scott Onstott)
Sam Kutler found the golden number in concentric circles.

The outer ring shows the 24 digit pattern(digital roots), the inner the 60 digit pattern(last number).
Lucien Khan rediscovered the 60 digit repeat pattern.
Fibonacci sequence hidden in ordinary division problem:
If you divide 1 by 999,999,999,999,999,999,999,999,999,999,999,999,999,999,999,999 (that’s 999 quattuordecillion), the Fibonacci sequence pops out.
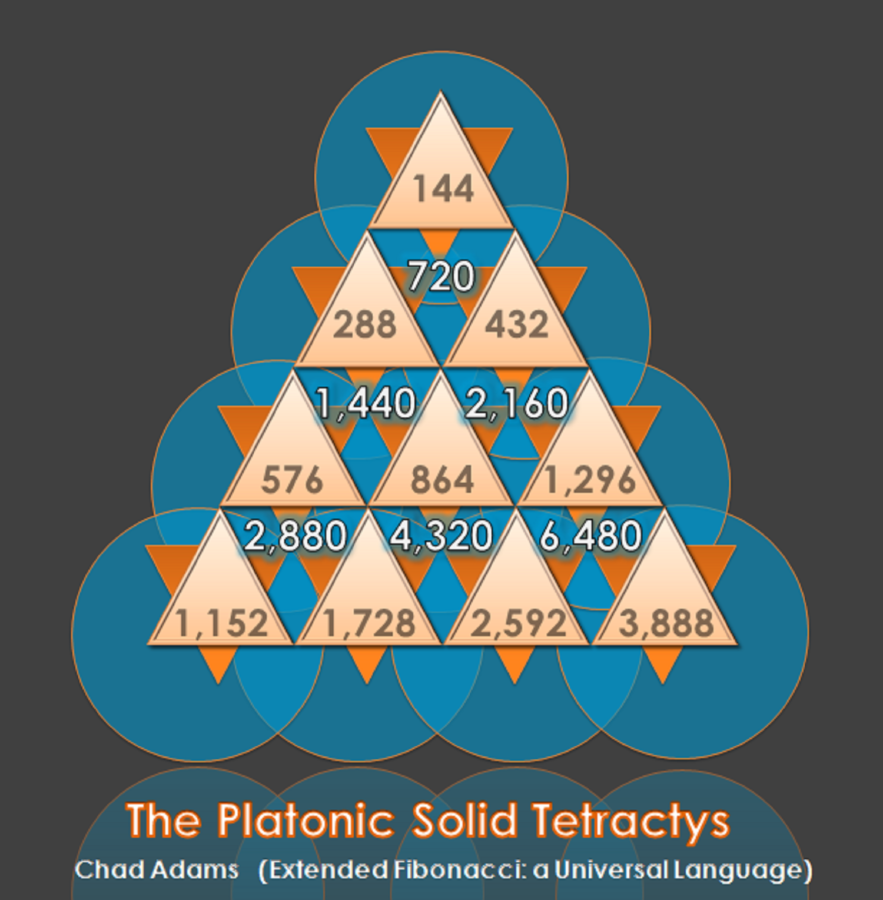
Chad Adam’s Extended Fibonacci Series
Like I said, you can start a Fibonacci sequence with any two numbers. If you begin with the numbers 1-9, some interesting things happen.
by Chad Adams
See how the numbers match up?
 back to the 60 digit pattern….
back to the 60 digit pattern….

The Fibonacci Sequence, starting at the 60th number in the sequence. See the pattern? Just look at the last digit in each number of the sequence. [after 8 is 3 because the last digit in 13(5+8) is 3]

Is it just me or is Colorado’s state seal illuminaty as all hell?
Don’t even go to Denver International Airport.
I did an article on Decoding the Dollar, and found the leaves on the left side of the buck encode the fib seq. with 2,3,5,8.
The name ‘Fi-Bonacci'(Man of cheer) comes from Leonardo’s old man who could always make the town laugh.

Leonardo de Pisa, the guy we call ‘Fibonacci”, actually had his own value for π, which was 864/275, or 3.141818181818…. (Phi = 1.618..)
I talk about this more here..
This is the number and sequence of numbers that God choose to use to structure all life and creation.





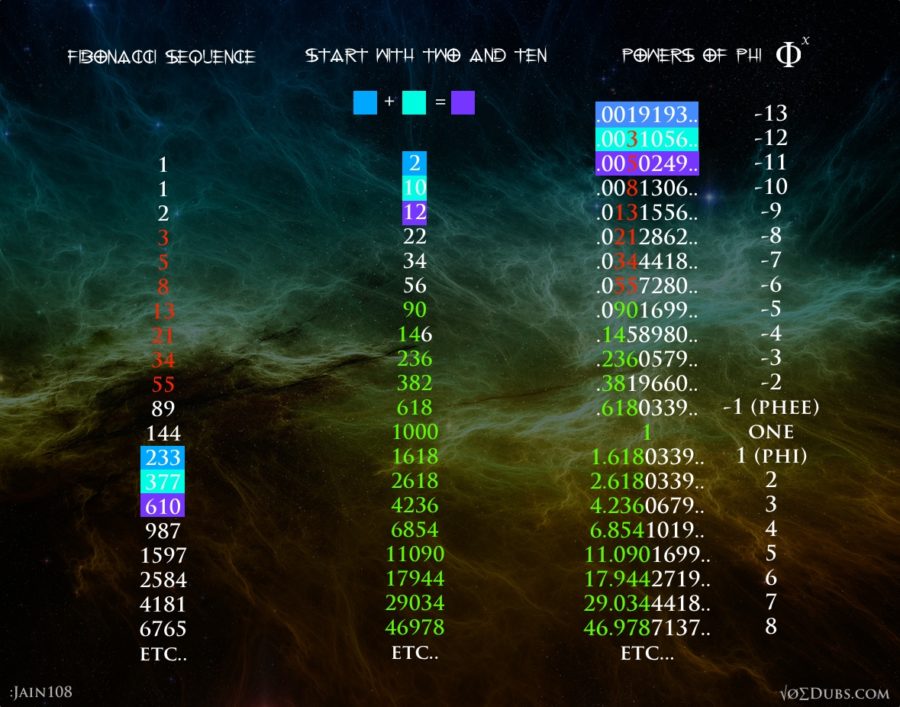
















Interesting pickup with the exponents of Phi within the first three decimal places (ie, some relationship to a 1000 divisor with only a very small offset).
If you take a look on my website, I explicate a strange coincidence between three types of Pyramids that I call the PPP-Pyramid … a trifecta that exists within a tiny threshold using the following constants:
1) 4/Pi
2) 14/11 (I call this ‘Perfect’ … Height divided by square base ‘radius’ [half pyramid base length])
3) Square Root of Phi
… these three pyramid designs fall in a range which is only 1/37th of a degree in total slope variance.
The fact that the number 1000 seems to connect Phi with the Fibonacci Sequence, has started my grey matter churning to attempt finding perhaps another simplified pyramid setup using the Fib.Seq. and the number 1000, which falls within the same range. I’ll let you know if I find a connection that is notable or revealing. It is strange that a base 10 number (1000) specifically allows the Fib.Seq. to be revealed in the Phi
The three PPP-Pyramid setups are listed in the following graphic:
https://aamichael666.files.wordpress.com/2014/11/the-three-ppp-pyramid-angles-phi-14-11-pi.png
… and a threshold examination using Polar to Equatorial Earth Radius in relation to Moon Radius is found in graph format for these three pyramid dynamics/constants in the following:
https://aamichael666.files.wordpress.com/2015/02/graph-visual-earth-moon-ppp-pyramid-analysis-at-all-latitudes-due-to-earth-flattening-pyramid-of-giza-lunar-standstill-shown.png
Absolutely brilliant 👏…. I am studying fibonacci for years and joedubs.com is brilliant.
Cathal Cregg.
Aw shucks, Thank you very much, I appreciate it!