The Platonic and Pythagorean Solids

The Platonic solids are 3-dimensional forms that nature uses to build herself.  The mental construct of reality seen in the form of geometry.     There are only five of them, naturally, since it is this (phi)veness that generates life as we know it.

The Platonic, Pythagorean, or Perfect Solids: Let’s imagine you are on the inside of a sphere and are asked to make a bunch of different three-dimensional forms using only straight lines that all touch the surface of the sphere.  You could make all kinds, an infinite amount actually.  But if every line had to be of equal length, and all the shapes made had to be the same, you could only make 5 different ‘perfect solids’.

The Platonic and Pythagorean Solids

These have been coined the ‘Platonic solids’, for his namesake, Plato.  Before his time they called them the Pythagorean solids because Pythagoras taught them in his mystery school.  There is no original ‘inventor’ of these solids because they were not an invention.  They were discovered, and it is debatable who first took notice.  Stone balls carved as these solids have been found in England and Scotland, dating back 5000 years.   It is very likely the ancients knew about these geometries.    As Manly P. Hall once wrote: “Mother Nature has shaken many civilizations from her back.”

These perfect solids are an intrinsic quality of our geometric reality.  There is esoteric knowledge hidden in the way Nature structures itself, the most obvious being that there are five of these forms that create reality.  There can be only five. You can prove this to yourself conceptually.  The following video illustrates

To make 3-D forms, first we need to start out with 2-D shapes. Two points on a piece of paper can’t make a shape, but three can, so the first shape is the triangle, the second is the square, and the third is the pentagon. The fourth shape is the hexagon but if you try to put a bunch of hexagons together to make a 3-D form it just doesn’t work.  Three hexagons makes a flat plane so you’re back to square one, pun intended.  Only triangles, squares, and pentagons can be combined three dimensionally to create ‘Platonic solids’.  Let’s just call them ‘perfect solids’ because it’s debatable who first discovered them, whether it was Plato, Pythagoras, the Scottish, or the Egyptians.

The Perfect Solids

The Tetrahedron – Fire

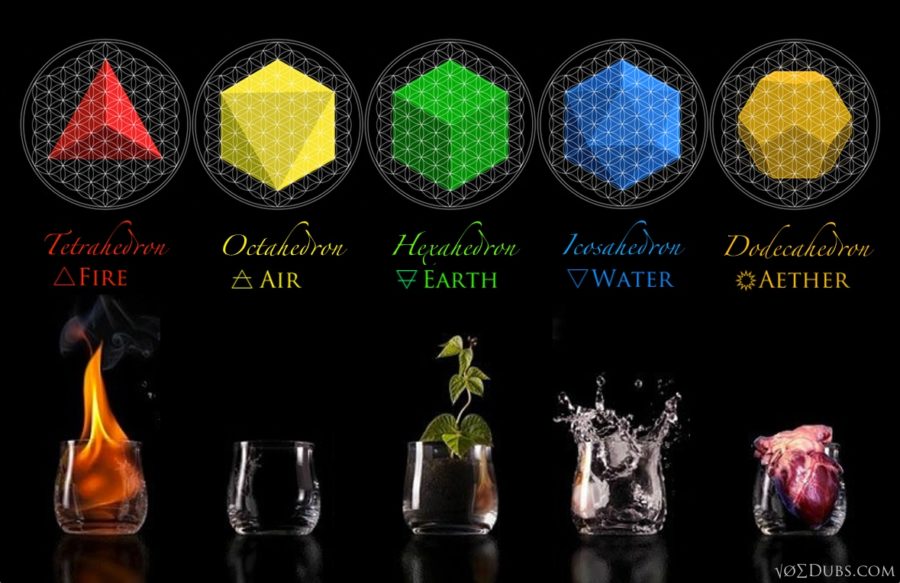
We can connect three equilateral triangles together to make a point. One more is required to fill in the other side for a total of four triangles. This is called the tetra-hedron, the first perfect solid. Tetra means four, and ‘hedron’ means sided. Plato associated this solid with Fire because its pointy ends mimic the stabbing sensation of a flame. Spicy food burns your mouth because of its tetrahedral composition at its molecular level.  At this point you’re sticking out your tongue trying to get more air, which leads us to our next solid, the Octahedron.

The Octahedron – Air

Four triangles fit together to make a pyramid.  Picture the great one at the Giza plateau.  However a pyramid is not a Platonic solid, since there’s a different shape at its base, a square.  If we took two great pyramids and put them together at their square base we would create the second most complex perfect solid; the octahedron, which symbolizes Air according the Plato and many other sources.

‘Now, the Greeks believed the world [material universe] to be composed of four elements–earth, air, fire, water–and to the Greek mind the conclusion was inevitable that the shapes of the particles of the elements were those of the regular solids. Earth-particles were cubical, the cube being the regular solid possessed of greatest stability; fire-particles were tetrahedral, the tetrahedron being the simplest and, hence, lightest solid. Water-particles were icosahedral for exactly the reverse reason, whilst air-particles,as intermediate between the two latter, were octahedral. The dodecahedron was, to these ancient mathematicians, the most mysterious of the solids; it was by far the most difficult to construct, the accurate drawing of the regular pentagon necessitating a rather elaborate application of Pythagoras’ great theorem. Hence the conclusion, as Plato put it, that ‘this (the regular dodecahedron) the Deity employed in tracing the plan of the Universe.’ (H. Stanley Redgrove, in Bygone Beliefs.)

‘Octa’ means eight. But, don’t let Octo-ber fool you being the tenth month of the year; Pope Gregory messed up the calendar 1500 years ago (I think..)  The first month is actually March, which makes a lot more sense.  In this regard, Sept-ember, Oct-ober, Nov-ember, and Dec-ember all fall on their respective etymologically numeric moon-ths. Sept means 7, Octo means 8, Nov means 9, and Dec means 10.  Maybe we should correct Pope Greg’s egregious mistake and fix the calendar.

The Icosahedron – Water

Now, back to geometry.  If we took five equilateral triangles and put them together at one point this would begin the construction of an icosahedron. Icosa, meaning twenty. We would have to add 16 more triangles to complete this solid.  This was associated with Water perhaps due to its ball-like shape, similar to a bead of water.  The icosahedron is the least stagnant and most spherical of the five solids.  If we took six triangles and put them together we would see that they form a flat surface, so this is no good. We can’t make any more of these perfect solids with triangles alone. Logically we have to go on to the next shape to make more perfect solids. Would you like to join us in this geometric adventure!? Be there, or be…… square.

The Hexahedron – Earth

The cube is the only perfect solid made of squares. Three squares join together to make a point. If we put four squares together at one point, we’d just get another larger square which is not three-dimensional.

Square has etymological roots in ‘quad’, which means four.

A cube is also called a hexa-hedron. Hexa means six. Hedron means sided. So we have a six-sided perfect solid made of squares. Plato associated the cube and the square with Earth. The sphere or circle would symbolize Heaven or the “One Mind” if you will. If there is a Creator and He or She started with a sphere, the cube might be the first choice to use since it models the most 3-D space out of any of the other perfect solids.

The Dodecahedron – Aether

The dodecahedron is the last of the perfect solids and is composed of pentagons. Dodecahedron means twelve sided. The twelve sides consist of pentagons which reflect the total number of perfect solids and encode the golden number which is inherent in all life. Three penta-gons (five-sided shapes) fit together at one point. Nine are added to complete the construction of the most mysterious and ineffable of all the perfect solids.

There are 360° degrees in a circle,  180° degrees in a triangle,  360° degrees in a square,  and 540° degrees in a pentagon.  With this information we can figure out the total angles in each of the solids.  This will prove to be useful when we are looking at distances in the cosmos, measured in ancient imperial units, like miles, furlongs, and feet.

The five ‘perfect solids’ total 14,400 degrees total in their angular measure. 144 is a very auspicious number, highly revered by the Ancients

The 3-D geometry of the universe is based on these perfect solids.  This knowledge was not invented, but discovered.  It’s inherent in all things.  This geometry is the armature or structure of the matrix that makes up our reality.

The first ‘Pythagorean Triple‘ – 3-4-5 Triangle

3,4, and 5 are the first numbers that combine to form the first so called ‘Pythagorean Triple’, which obeys the rule ‘A squared plus B squared equals C squared’ (See…high school geometry did come in handy after all). The physical sizes of Earth and Moon encode the 3-4-5 triangle, which itself also encodes Phi, and therefore encodes life.  Earth and Moon are not alone, however. We must include Sun in the trinity.  The spelling of their names, in English, is a clue to unraveling the Pythagorean Triple Mystery.

 S u n(3), M o o n(4), E a r t h(5).

If the Moon was pulled next to Earth the dimensions would create two 3-4-5 triangles on either side.  The squares around Earth and Moon are 11 and 3, respectively.

Like our five senses there are five Platonic solids, each of which is made up of shapes that have 3,4, or 5 sides. The tetrahedron is made up of 4 triangles(3-sided), the octahedron, 8 triangles. And the icosahedron has 20 triangles. The cube is composed of 6 squares(4-sided) and the dodecahedron is made of 12 pentagons(5-sided). Three-Four-Phive is reflected in the way nature structures itself, based upon the simplest 3-D geometries in our reality.

Can you see the three-four-fiveness? Both in the shapes and how the shapes meet? Perhaps we should call them Pythagorean solids since they encode his famous 3-4-5 triangle?

In addition the Platonic solids also encode 3-4-5 in the way the shapes fit together. The tetrahedron, cube, and dodecahedron have three shapes at their corners. The octahedron, four. And the icosahedron has five shapes coming together at every point. Again, the Three-Four-Phiveness, in the way Nature structures itself.

The angles of the perfect solids display a pattern that is broken with 5040, which is the mean radii of both Earth and Moon in miles. The angles of the octahedron has no place on this triangular grid.

The harmony of the world is made manifest in Form and Number, and the heart and soul and all the poetry of Natural Philosophy are embodied in the concept of mathematical beauty.”  -D’Arcy Wentworth Thompson

A physics professor at the University of Chicago by the name of Robert Moon developed a model of the Periodic Table of Elements showing the Platonic Solids as the pattern of the neutrons and protons in the nucleus of each element.

Walter Russell originally made this chart which maps the elements in a spiral.  Later somebody added in Moon’s discoveries.

“Geometry is knowledge that appears to be produced by human beings, yet whose meaning is totally independent of them.” -Rudolf Steiner

A Study of the angles of the solids, and how they stack up with each other

“Pythagoras was one of the first teachers to establish a community wherein all the members were of mutual assistance to one another in the common attainment of the higher sciences. He also introduced the discipline of retrospection as essential to the development of the spiritual mind. Pythagoreanism may be summarized as a system of metaphysical speculation concerning the relationships between numbers and the causal agencies of existence.” -Manly P. Hall

“Numbers are the Highest Degree of knowledge. They are knowledge themselves” – Plato

“While spinning his amazing tales about the nature of the universe, often in the same words, Plato gave a scientific account of creation with mathematical formulas for the benefit of his initiated readers, His Creator proceeded in the same way as God in the Apocrypha (Wisdom 11:20), of whom it is said, “Thou hast ordered all things in number and measure and weight.” As the first stage in his plan to make all things as like himself as possible, he reduced primeval chaos to order by setting numerical proportions between its elements. These were symbolically named earth, water, air, and fire. The Creator fabricated the universe form the most and the least corporeal of the elements, earth and fire, linking them by placing water and air as mean terms in a geometric progression that bound them all together.

“In bringing order to the universe, the Creator gave geometric forms to the atoms of which the four elements are separately composed. The simplest of the five regular figures of solid geometry, the tetrahedron, is the shape of the fiery atom, fire being the most volatile of the elements and the tetrahedron the least stable of the solids. The most stable, the cube, forms the atoms of earth, the octahedron is the form of air, and the twenty-sided icosahedron of water. Compounded together in various proportions and of different sizes, the atoms of the four elements make up the entire physical universe, interacting with human senses to produce appearances and feelings. The fire atom, for example, which has the sharpest angles and edges, causes the sensation of heat by cutting and stinging.

The Platonic solids, so called because of their appearance in Timaeus, are there defined as “solid figures which divide the surface of a circumscribed sphere into equal and similar parts.” There are only five of them, those that Plato related to the four elements together with the dodecahedron, which, he said, “was used by God for arranging the constellations of the whole heaven. ” Elsewhere (Phaedo, 110), Plato compares the earth to a dodecahedron, saying that when seen from above, it resembles “one of those balls which are covered with twelve pieces of leather.”

In the course of their interactions and collisions, atoms are constantly being broken up, whereupon their constituent parts come together again, sometimes forming different bodies and being transformed from one kind of atom into another. These transformations occur between the atoms of fire, air, and water because the solids that represent them, the tetrahedron, the octahedron, and the icosahedron, are made up superficially of the same kind of triangle, the equilateral. When the atoms are broken up, the triangles into which they fall reassemble to make new atoms of either fire, air, or water. The cube, which is the solid representing earth, cannot, however, be divided into equilateral triangle’s; the paired triangles that make up each of its sides are isosceles. When broken up, the earth atoms do not therefore transform into other types of atom but instead reform themselves as cubes.

The model of the physical universe, as Plato described it, would be a perfect, all-inclusive sphere, containing every type of proportion as represented by the five regular figures of solid geometry. Such a model was constructed in the 16th century by Johannes Kepler, demonstrating celestial mechanics by a combination of solids containing containing the spheres of planetary orbits, all within an outer sphere beyond the fixed stars. The universe, however, is no mere mechanism but a living organism by virtue of its soul. According to Plato, the world soul was the Creator’s primary work, and in Timaeus, 35-36, he gave the formula of its composition.”

-John Michell, The Dimensions of Paradise

artist: I am an Astronaut           song: Winter Solstice          album: Aether Experiment