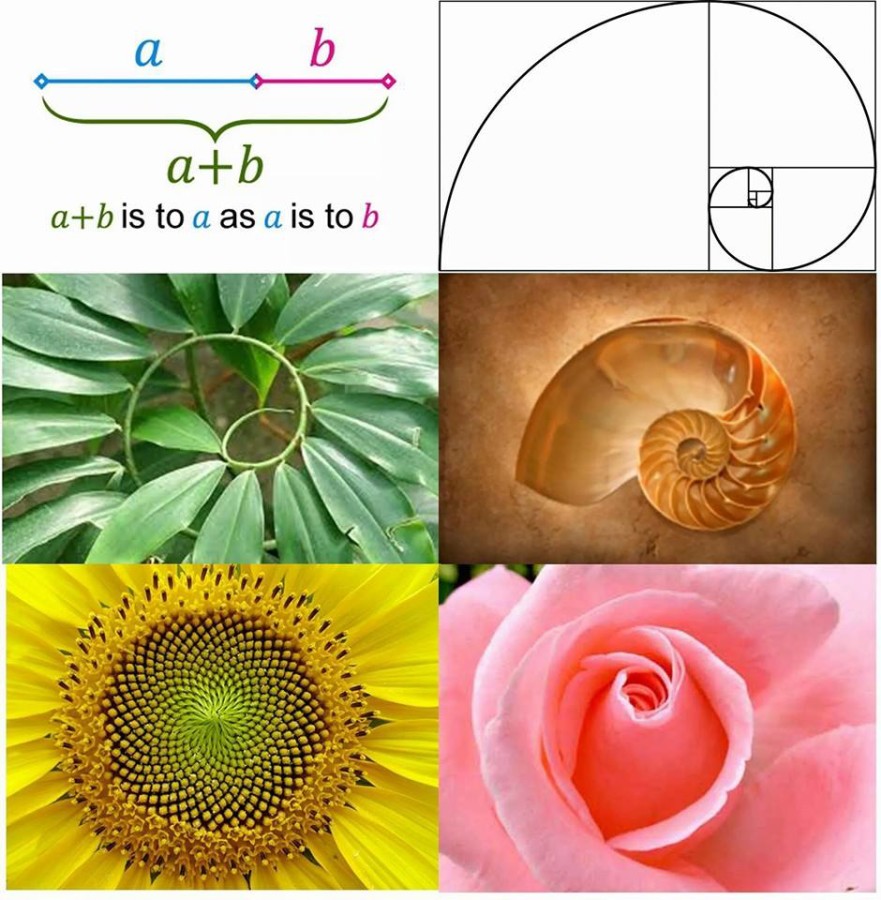
There is one proportion that is divine. A ratio of two numbers, the simplest of which being one. Paired with unity, there is only one number that is capable of fractal expansion and contraction. By using the four operations of mathematics: addition, subtraction, multiplication, and division, this golden number (1.6180339..) is the key to understanding fractality, holograms, life, and the nature of reality. This is the number (or ratio) the universe uses to become more aware of itself.
The divine proportion is the only mathematical ratio that the Universe needs to intelligently design all life. Phi, aka the Golden Ratio, is a truly divine number. It’s quite a phinomenon. Along with unity this number creates the perfect proportion. Let’s demystiphi some of the more esoteric geometric concepts attributed to this golden proportion.
Phi is found everywhere and yet nobody talks about it. It’s missing from the calculator. Its importance remains hidden even from the most hard-core math geeks. Most mathematicians have heard of it, but the mystery surrounding its existence is ineffable, and the complete understanding of it, perhaps insurmountable.
The Golden Ratio is a universal law in which is contained the ground-principle of all formative striving for beauty and completeness in the realms of both nature and art, and which permeates, as a paramount spiritual ideal, all structures, forms and proportions, whether cosmic or individual, organic or inorganic, acoustic or optical; which finds its fullest realization, however, in the human form.
–Adolf Zeising
“Geometry has two great treasures: one is the theorem of Pythagoras; the other the division of a line into extreme and mean ratio. The first we may compare to a measure of Gold; the second we may name a precious jewel.” -Johannes Kepler
This ratio is also known as the divine cut, golden mean or golden section. Other names include Phi, extreme and mean ratio, medial section, divine proportion, divine section, golden proportion, golden cut, and golden number.
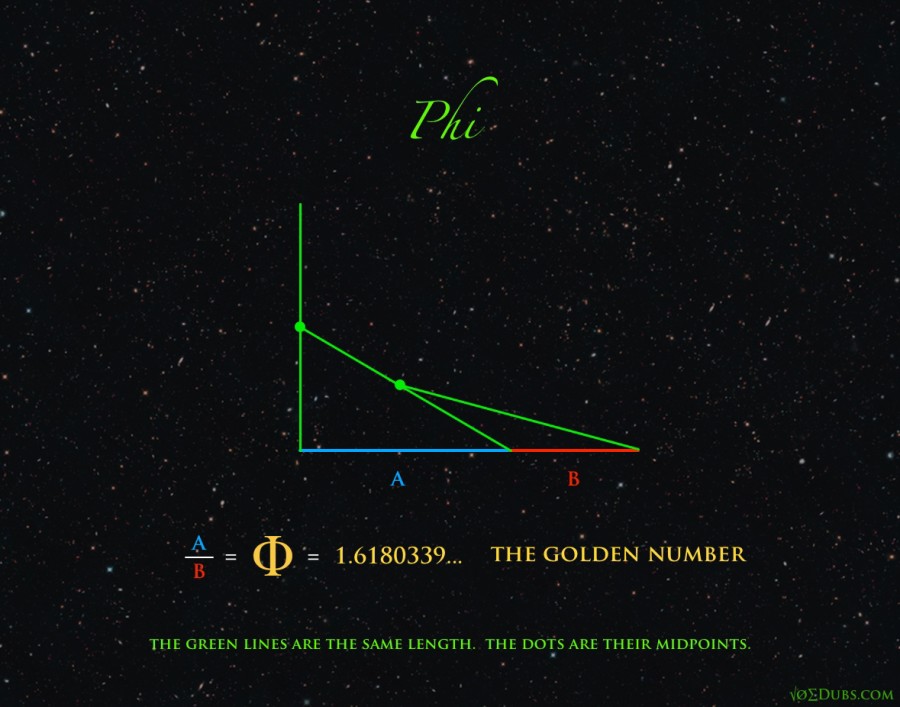
A straight line is said to have been cut in extreme and mean ratio when, as the whole line is to the greater segment, so is the greater to the lesser. -Euclid
With the golden ratio the whole line is to the large line; as the large line is to the small line.
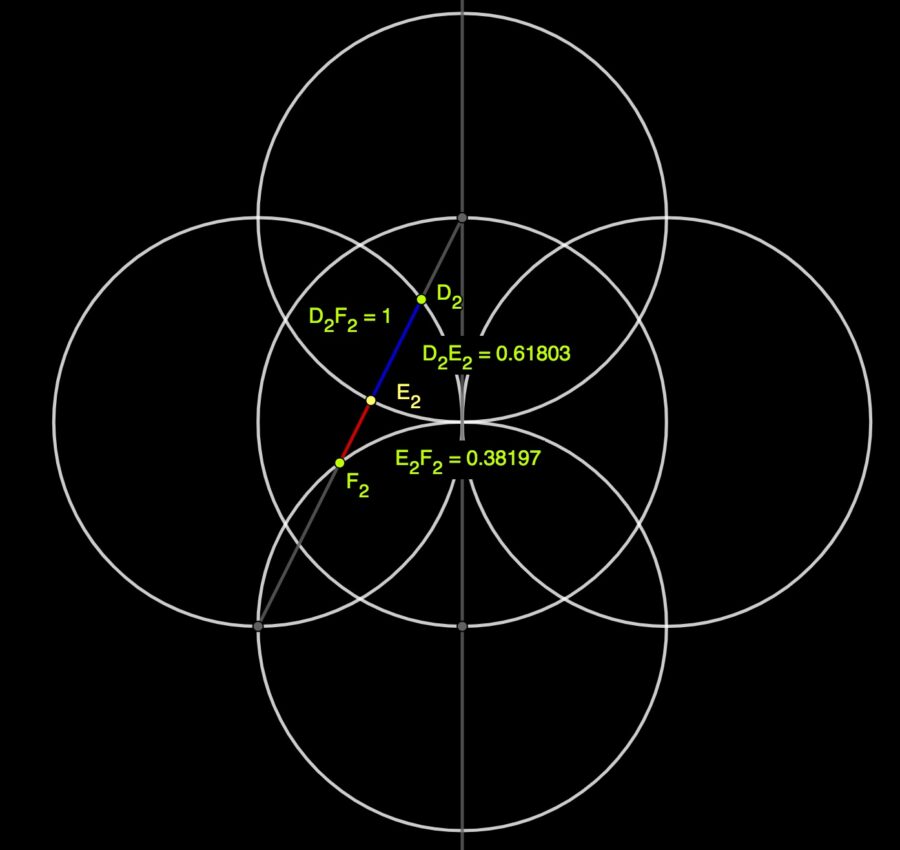
If we were to take a line and divide it, there is only one place where the two separate lines would be in golden proportion with each other. One side would be 1 and the other, 1.618. Or one side would be .618 (1/ø) and the other 1. This is the only ratio that works this way. It is the only ratio in geometric and mathematical progression. It’s perfect!
Phi (1.618..) and phee(.618..) are the numbers of fractal expansion and contraction. Like male and female, these two energies spiral around unity, creating life as we know it.
The powers of Phi work just like the Fibonacci sequence. 1 + Phi = Phi squared. Phi + Phi squared = Phi cubed, and so on. This is the only number that does this. Phi is the only number where its square is exactly 1 number larger. It’s also unique in that its reciprocal (1/Phi) is one less than Phi, which we’ll call phee (0.618..)

Phi is the only number that can do this. Perfect fractal geometric and mathematic expansion and contraction
According to NASA the physical sizes of Earth and Moon reflect the divine proportion.
Jonathan Quinton made this video that beautifully displays the nature of the Golden Ratio.
Geometric Constructions of the Divine Proportion
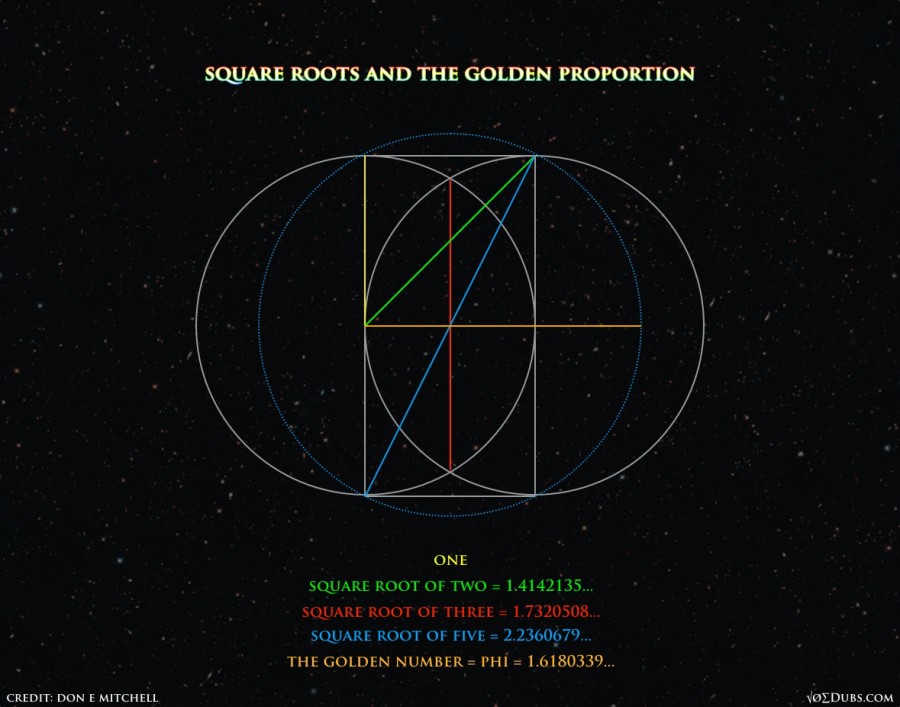
Phi arises out of simple geometries. Take three lines of equal length and at their midpoints arrange them in this manner. Phi originates from the square root of five. (ø = (√5 +1/2). Being the number of life, Five is closely connected to Phi. Even here the midpoints are .5 their full length. Maybe we should spell 5 “Phive“.
The square root of five is the cross section of the double square as seen above in blue. The square root of 4 (or 2) is the length of the double square. The square root of three comes from the vesica piscis. If the circle’s radius is one, the vertical length where they connect is the square root of three. Phi (seen in gold) arises from the relationship shown. The radius of the blue circle is the square root of five divided by two, or 1.180339… which is also Phi minus one half. The diameter of the blue circle is the square root of five.
This construction was developed by George Odom and published in American Mathematics Monthly in 1983. A circle with the simplest shape inside it reveals golden ratios, both in linear and curvilinear representations.
“Phiangle” further expanded upon by Michael S. Schneider
Starting with the circle on the right, divine proportions are derived in this way.
A very basic construction of Phi. The arc of the circle is needed to create it.
Phi constructed from three circles. John Michell gets credit for this one.
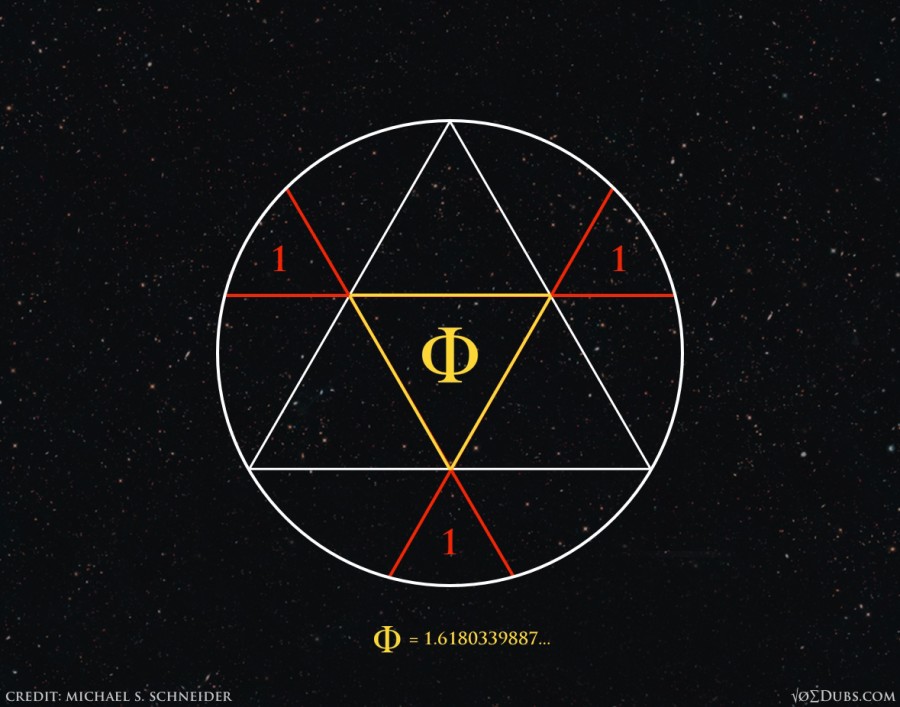
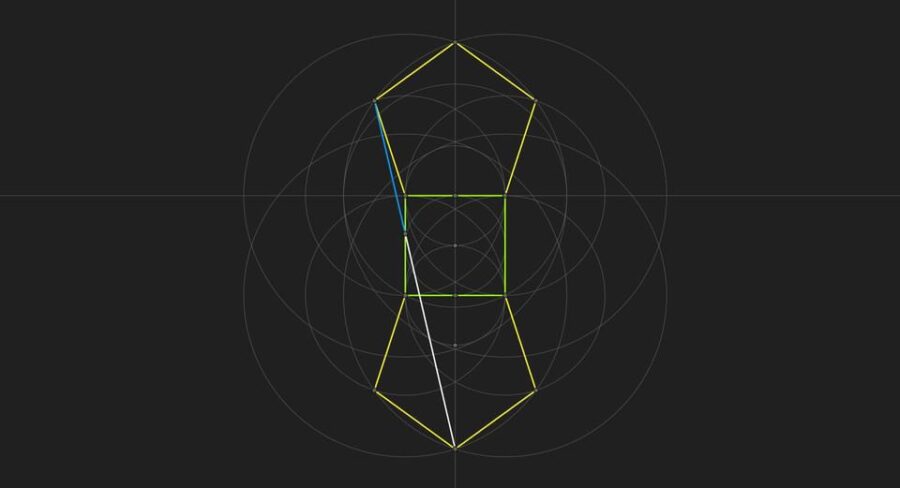
Phi springs to life from the geometry of five. Golden proportions are ubiquitous in the pentagons and pentagrams. It is this “phiveness” that brings Phi to life.
Robert Lawlor, in his book ‘Sacred Geometry’, shows Phi proportions with a circle split into 5×2 sections.
“ Where plants have five-fold patterns, a consideration of their souls is in place. For patterns of five appear in the regular solids, and so involve the ratio called the Golden Section, which results from a self-developing series that is an image of the faculty of propagation in plants. Thus the flower carries the authentic flag of this faculty, the pentagon.”
– Johannes Kepler
In 2006 Bengt Erik Erlandsen showed that Phi can be represented simply in this way. Whenever you drive down the street you are subconsciously experiencing golden ratios. Again, with the appearance of five. This time the circles on the top and bottom are cut in half (or .5)
In 2002 Kurt Hofstetter published this construction in Forum Geometricorum. The vesica piscis (the two smaller circles) in combination with circles around the “bladder of the fish”, created phi proportions. He struck gold on this one.
Constructing the pentagram using circles and phi ratios: Starting with the green square, circles are extended out which define the points of the pentagram. The radius of the green circle is 1, the yellow 1.618.., and the magenta 1.23..
Vesica Phiscis, well not quite. The name may be a play on words, but the golden proportion inherent in this geometry is forever accurate. Phi seems to come up a lot analyzing circles. It may seem odd then that Phi is not encoded into the relationship of a circle’s circumference to its diameter, (π). Pi is well-known. It even has its own day. The day, might I add, this website was launched.
Why then, is Phi not encoded into the ratio known as Pi? Well some mathematicians think it is. My Australian mathemagician friend Jain108 has concluded that the ratio of a circle’s circumference to its diameter IS linked to Phi. He goes on to show that π = 4/√ø. Four divided by the square root of Phi, which is a little over the accepted value today. (3.1446..) More on this in an upcoming article.
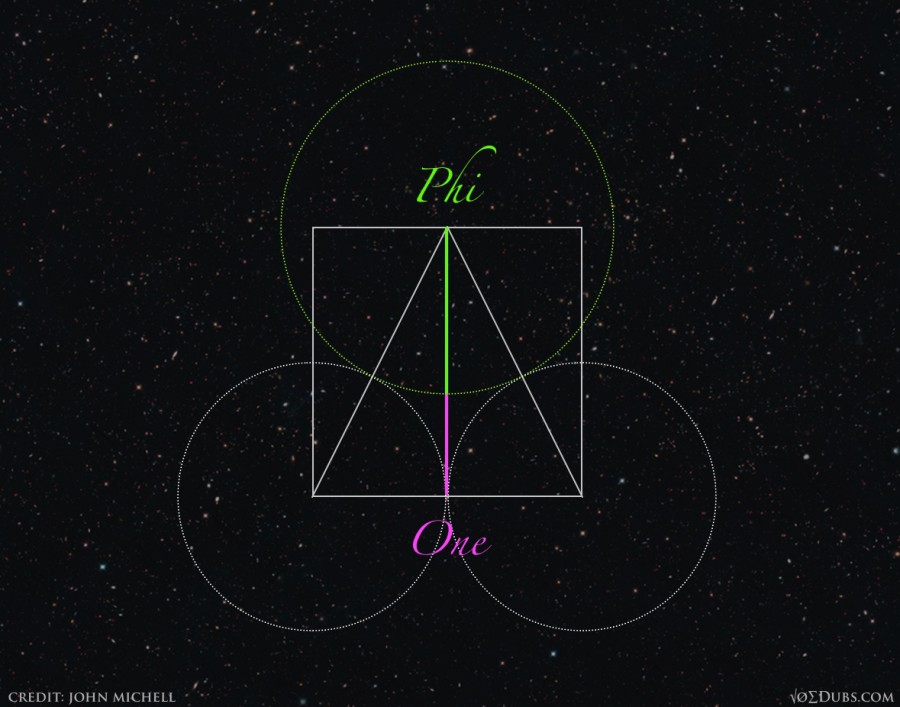
The green and red circles are in golden proportion with each other. The blue square and circle have the same perimeters. Thus Phi encodes the solution to “squaring the circle”.
Blue is to Red; as Magenta is to Green. Isn’t that phinomenal?!
The Pentagram and the many manifestations of Phi. One is always needed in between to transition from the higher powers of Phi into the lesser. These golden numbers Phi and phee, 1.618 and .618, are never without the presence of ONE.
My friend Rhuben Neal made this construction. If the diameter of the blue circle is 1, the diameter of the golden circle is Phi cubed.
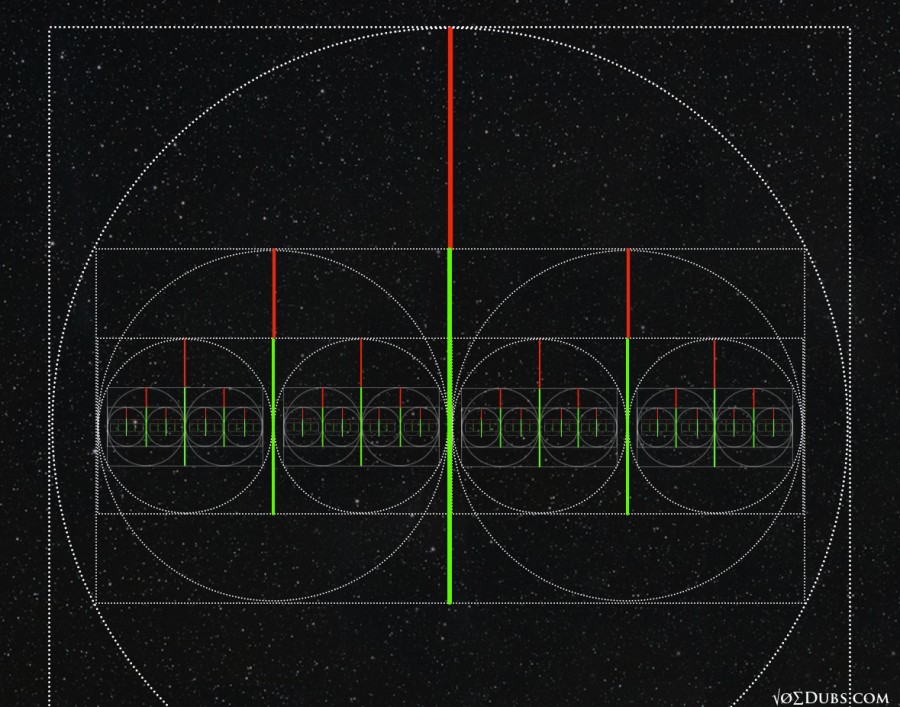
Fibonacci Circles. Original construction by Sam Kutler.
Gary Meisner’s website GoldenNumber.net explores Phi in depth.
Phee Phi Pho Phum, I smell the blood of the Golden One.
“Neglect of mathematics work injury to all knowledge, since he who is ignorant of it cannot know the other sciences or things of this world. And what is worst, those who are thus ignorant are unable to perceive their own ignorance, and so do not seek a remedy.”
― Roger Bacon, Mathematics
This simple drawing reveals a lot, but like Hermes put it, The lips of wisdom are closed, except to the ears of Understanding. Or in this case, eyes. The length of the square, in green, is one. The hypotenuse in magenta is √5 or 2.2360679.. The blue line is double the green. In red is ‘phee’ sometimes labeled lowercase phi. In Gold, Phi. The circle, as it seems in these drawings, is vital to the existence of this divine proportion.
The double square encodes Phi with the help of the circle. If the length of one square is one, the double-square’s perimeter is six, and the cross section(in magenta) is the square root of five. This relationship of 5 to 6 is encoded into the fabric of our reality. Five is the number of life, and Six the number of structure. These two archetypes combine to construct our numerical universe.
”He who joins the hexagram and pentagram has solved half of the sacred secret.” –Eliphas Levi (19th century magician)
Another phi construction ^ via George Leoniak
Elliot McGucken discovered a Phi relationship between the 3, 4 & 5 sided polygons^ https://www.cut-the-knot.org/do_you_know/Elliot2.shtml
^Here shows the edge relationships of regular polygons from a triangle to the hexagon inscribed in the same size 1 radius circle. [Credit to Hunter Hughes]
The following construction was made by George Leoniak
^ constructed by Hunter Hughes
“Some of the greatest mathematical minds of all ages, from Pythagoras and Euclid in ancient Greece, through the medieval Italian mathematician Leonardo of Pisa and the Renaissance astronomer Johannes Kepler, to present-day scientific figures such as Oxford physicist Roger Penrose, have spent endless hours over this simple ratio and its properties. But the fascination with the Golden Ratio is not confined just to mathematicians. Biologists, artists, musicians, historians, architects, psychologists, and even mystics have pondered and debated the basis of its ubiquity and appeal. In fact, it is probably fair to say that the Golden Ratio has inspired thinkers of all disciplines like no other number in the history of mathematics.” -Mario Livio
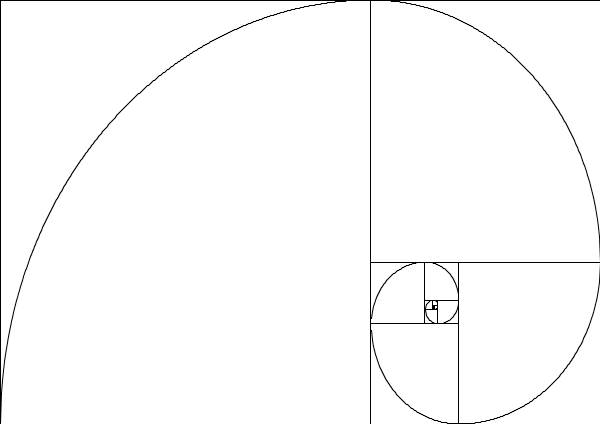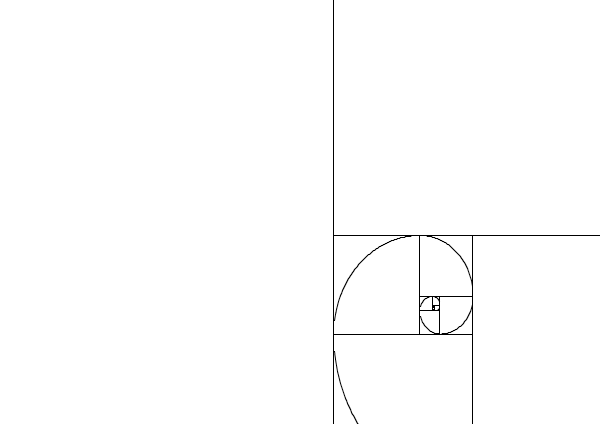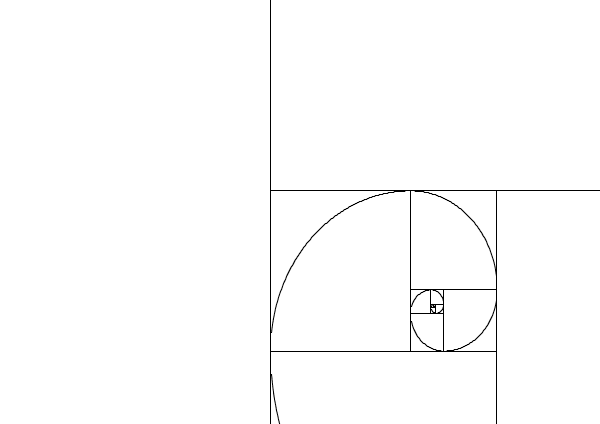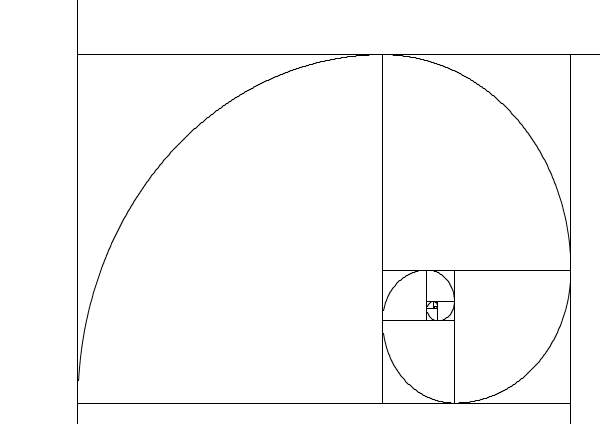
Patterns in the Fibonacci Sequence



































great article…have been fascinated by phi for quite a while…..found some spooky decimal mirroring in the positive and negative powers of phi:
The decimal expansion of the negative even powers is one less the decimal digits of the corresponding positive power, so the expansion of the even powers looks different, and the mirroring of the digits seems to disappear in them. The successive powers of phi produce therefore a pattern in which they alternately display that reflection and then hide it again, just as the moon alternately reflects the light of the sun and then does not. This striking pattern is easy to find, and any curious ancients who explored the powers of this constant could easily have discovered it.
Digit sequence symmetries
in positive and negative powers of phi:
phin
phi1 = 1.61803398875
phi2 = 2.61803398875
phi3 = 4.23606797750
phi4 = 6.85410196625
phi5 = 11.09016994375
phi6 = 17.94427191000
phi7 = 29.03444185375
phi8 = 46.97871376376
phi9 = 76.01315561752
phi10 = 122.9918693812
phi11 = 199.0050249987
phi12 = 321.9968943800
phi13 = 521.0019193789
1 / phin
1/phi1 = 0.61803398875
1/phi2 = 0.38196601125
1/phi3 = 0.23606797750
1/phi4 = 0.14589803375
1/phi5 = 0.09016994375
1/phi6 = 0.05572809000
1/phi7 = 0.03444185375
1/phi8 = 0.02128623624
1/phi9 = 0.01315561752
1/phi10 = 0.00813061875
1/phi11 = 0.00502499874
1/phi12 = 0.00310562001
1/phi13 = 0.00191937894
Thanks, yes Phi is phascinating isn’t it. I know the fibonacci sequence is found in the decimal expansion of the reciprocal of 89, but have never seen anything like what you’re suggesting. Very interesting!
Very interesting concerning Golden Ratio.
Furthet attach links relating it implications of its Golden Root.:
http://www.stefanides.gr/pdf/2012_Oct/PHOTO_12.pdf
http://www.stefanides.gr/pdf/2012_Oct/PHOTO_09_PCST_GEOMETRY.pdf
http://www.stefanides.gr/pdf/GOLDEN_ROOT_SYMMETRIES_OF_GEOMETRIC_FORMS_by_Panagiotis_Stefanides.pdf
Regards from Athens,
Panagiotis Stefanides
http://www.stefanides.gr
Thank you, Panagiotis. This looks very interesting.. 3.1446.. I recognize that number right away!
“Hidden harmonies are more than obvious.”
Heraclitus
https://medium.com/@ASYNSIS/hidden-harmonies-are-more-than-obvious-1eb24148fa93
Speaking of those ideal geometric forms, they’re also flows: they’re signatures of thermodynamic processes, I’ve recently shared on TED.com how the golden ratio can also be seen as a temporal signature (an asymptotic convergence, to be precise), of dynamical systems universality, of how nature evolves emergent complexity most robustly, adaptively and rapidly.
In my talk, I also share a process I call “Form Follows Flow” (since all functions are ultimately information flows – energy-matter patterns), how the thermodynamic flows described by Adrian Bejan’s Constructal law of Design in Nature & Culture are epitomised by the ideal, optimal forms of the Asynsis principle.
But really, looking exclusively at only Spatial interpretations are so last century anyway. We need to look at what nature is doing in Time.
And does that also mean the entire repertoire of plant phyllotaxis is an illusion?
Plants are telling us something, that we need to think harder about the Temporal, about energy and growth, as Alan Turning did in his last research on the Fibonacci series, phyllotaxis and morphogenesis before he tragically died at an early age.
We need to look at both the animate and inanimate, at the dynamical and space-time, not just the frozen and static. It seems the way nature works is to manifest the golden ratio not as a spatial fossil, rather an optimal, analogical temporal energy flow signature. An efficiency (& beauty) constant.
All this current argument is only over the residue or sedimentary forms of these dynamical flows – so we are missing half the picture (as I’ve been sharing since first publication in AD Magazine, based on Masters studies in Architecture & Computer Science at the University of Westminster, London).
What the sciences of complexity and dynamical systems have been revealing recently is that the golden ratio is one of several optimal, analogical geometric signatures of how nature evolves emergent complexity most easily (for the least energy-resource cost, with vital sustainability-promotion consequences) – over time.
It’s a dynamical behaviour, a verb not a noun.
It’s not the phone number, but the very action of dialing.
Here’s how I Tweet it:
#Asynsis #DaoOfDesign on #TED at #TEDxWanchai #HongKong.
A New, Extremely Lean, Mean (#Design) #TheoryOfEverything
The very latest examples from across a wide range of the sciences (including E8 & ER=EPR #Universality), are shared here (so please enjoy!):
#AsynSoφia
https://www.facebook.com/AsynSo%CF%86ia-202383966558282/
Entropy begets Design – QED:
https://asynsis.wordpress.com
THank you! Allow me to check this out and get back to ya
-joe
Joe, I have more email material compiled for you.
The one I have been communicating with revealed Herself to me the other day.
I have some law-defying work to present to you, if you may.
Sincerely, He Whom Is Able By Thoth, Not By Birth.
He Whom Is Able By Thoth, Not By Birth in English Gematria Equals: [2106]
“He Whom Is Able By Thoth, Not By Birth Is not in our gematria database”
ABCDEFGHIJKLMNOPQRSTUVWXYZ in English Gematria Equals: [2106]
The Radius Of Mars: 2,106 Miles
Mars is the ‘Planet of War’. 2016 is 2106 rearranged on binary terms.
2016 is the year for WW III. The war between the eagle and the bear, (jachin and boaz) will be the stepping stone for the NWO. The date the Illuminati was born (May 1st 1776) is the rabbit hole to everything we know to exist — speaking of rabbit hole, CERN is a rabbit hole that is penetrating outside of our rabbit hole to communicate with the returning entity on the other side. Want to know more about what really is going on underneath a surface of apparent order? Connect the date of Illuminati to the planets, light, gravity, our human body’s composition and the various elements & principles of reality. If you really want to know the big picture, start realizing that the distance between America and Russia between their major monuments and architects are mathematically superior to all things in this temporary physical manifestation.
All roads lead to Rome, then snatch your soul.
Joe, I put together a video that should help you understand what Pi is.
Pingback: Square Roots & Stuff
one more picture https://www.facebook.com/photo.php?fbid=1553628388267112&set=a.1405456039751015.1073741827.100008598961183&type=3&theater
a link about Pi sry in french http://www.neotrouve.com/?p=381
Pingback: The Golden Ratio (phi) – Symbolism of Mathematics