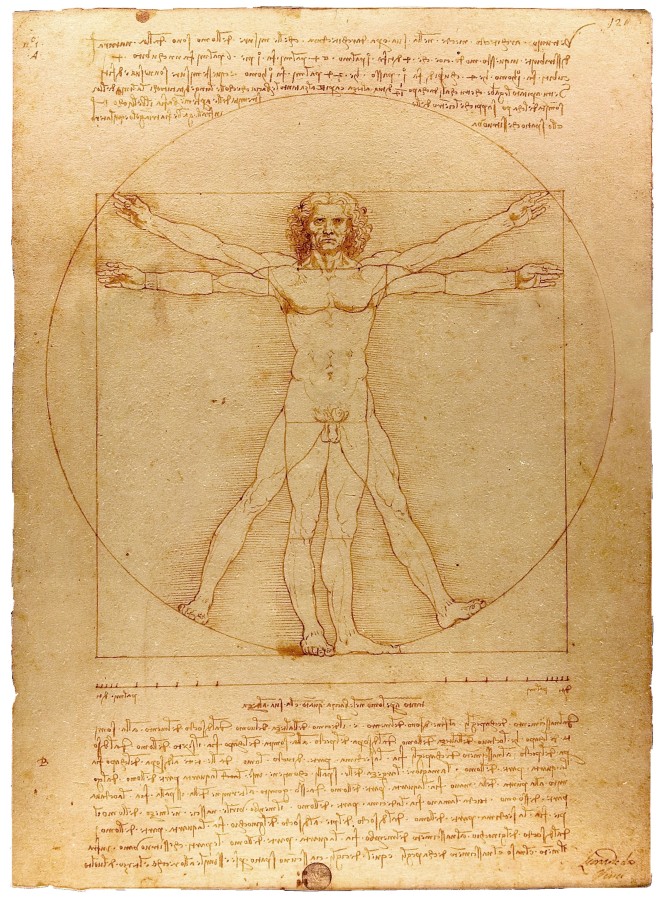
Leonardo da Vinci’s famous depiction of Vitruvius encodes geometrical gnosis that’s hidden deep beneath the collective unconscious. Let’s man handle these esoteric concepts and bring light to where there is darkness
Leonardo was familiar with the golden ratio and modeled Vitruvian’s square and circle off it.
The square and circle Leonardo used are of Phi ratios. 1 : 1.6180339…
It turns out that this Phi ratio is almost exactly smack dab in the middle of the two solutions of ‘squaring the circle’, that is drawing a square that is commensurate to a circle, either by having equal areas, or equal perimeters ( or lengths).
If the circle has a radius of 1, and thereby a diameter of 2, a square with the same area has a width of the square root of π. A square that has the same perimeter as the circle has a width of π divided by 2.
The square with the same area as the circle (in blue) is wider than the square that has the same perimeter (in green). The square Leonardo used (in gold) is just about half way between these two different geometrical formulas. Since the human body, as well as all life, utilized phi ratios in its construction, Leonardo decided to use this prolific ratio for the width of Vitruvius’ square.
The circle extends 12.8379% beyond the width of the blue square (equal areas).
The circle extends 27.32395% beyond the width of the green square (equal perimeters).
The golden square, which is in Phi proportion, extends 23.60679% beyond the width of the circle. (The square root of five is 2.2360679..)
The golden square lies in between these two geometrical solutions. But isn’t not perfectly in the middle. If you want to say it is, well that would only be a 97.08% correct statement.
The circle extends almost exactly 20% (20.0809%) beyond the mean of the blue and green squares (not shown). What’s interesting is that this is the ratio of 6/5, which is ubiquitous in the study of ancient cultures and geometrical/mathematical esoterica. This is a 99.93% accurate correlation, using the traditional value of π. (3.14159265..)
“I see 6/5 more as a resonance built into the architecture of the universe.” – Scott Onstott
1.2 x 1.618.. x 1.618.. = 3.1415..
”He who joins the hexagram and pentagram has solved half of the sacred secret.” –Eliphas Levi (19th century magician)
The Pentagon and Hexagon – The Microcosm and Macrocosm
The Snowflake and the Flower (six and five)
“ [The existence of pentagons and hexagons] doth neatly declare how Nature Geometrizeth and observeth order in all things.”
– Sir Thomas Browne
There’s two ways to ‘square the circle’. Equal perimeters (in green) or equal areas (in red)
“Squaring the circle” is the alchemical process of transferring an airy concept from the mental plane to the physical dimension so that objective conception and birth become a demonstrative reality. – Dr. John Munford
Vitruvius laid upon the Great Pyramid, and Earth-Moon system, both of which square the circle (perimeters)
“The squaring of the circle is a stage on the way to the unconscious, a point of transition leading to a goal lying as yet unformulated beyond it. It is one of those paths to the centre.” – Carl G. Jung
We humans are made of phi ratios
Leonardo Da Vinci’s ‘Vitruvian Man’ displays Man within a circle and a square. The square he used is a consolidation, a duality of two distinct solutions to ‘squaring the circle’, or obtaining the unobtainable. We can never truly square the circle due to the nature of π. Did Leonardo hide the solution of this ancient philosophical quandary by suggesting that man is the mean between two transcendental impossibilities?
“Squaring the circle was a problem that greatly exercised medieval minds. It is a symbol of the opus alchymicum, since it breaks down the original chaotic unity into the four elements and then combines them again in a higher unity. Unity is represented by a circle and the four elements by a square. The production of one from four is the result of a process of distillation and sublimation which takes the so-called “circular” form: the distillate is subjected to sundry distillations so that the “soul” or “spirit” shall be extracted in its purest state. The product is generally called the “quintessence,” though this is by no means the only name for the ever-hoped-for and never-to-be-discovered “One.” It has, as the alchemists say, a “thousand names,” like the prima materia.”
— Carl Jung
Geometrical construction of the Vitruvian Man by Leonardo da Vinci
Discover more from JoeDubs
Subscribe to get the latest posts sent to your email.
INTERESTING!
PLS REF:
http://gallery.bridgesmathart.org/exhibitions/2014-bridges-conference/panagiotis-stefanides
http://www.stefanides.gr/pdf/BRIDGES_2014_PANAGIOTIS_STEFANIDES.pdf
Thank you Panagiotis, Right back at ya!
I LOVE YOUR BLOG, FACEBOOKPAGE, JUST ALL OF IT!!!!!!
Thank you 😉
There is pentagon and hexagon in human body. Then……………… put on hand on belly button (hexagon) , and chest look like pentagon. (I don’t know English well)
The Square and circle of the human figure:
• Remember again that the distance between the human individual’s outstretched arms is equal to the height of the human individual from the top of the head to the bottoms of the feet. Because the distance between the outstretched arms of a human individual is equal to the total height of a human individual from top of the head to the bottoms of the feet a square can be drawn around the physical figure of the human individual.
A circle with a circumference equal to the perimeter of the square that has width equal to the height and outstretched arms from fingertip to fingertip of the human individual can be created if the width of the square is used as the second shortest length of a Kepler scalene triangle and the diameter of the circle with a circumference equal to the perimeter of the square that has a width equal to the height and outstretched arms of the human individual is used as the longest length of the Kepler scalene triangle. The longest length of Kepler scalene triangle divided by the shortest length of a Kepler scalene triangle results in the Golden ratio of: 1.618. Only 2 of the 4 corners of the square can touch the circumference of the circle that has a measure equal to the square’s perimeter with this version of squaring the circle with the human figure. The shortest length of the Kepler scalene triangle has 2 points that both touch the circumference of this circle that has a measure equal to the perimeter of the square with a width that is equal to both the breadth and height of the human figure. The shortest length of the Kepler scalene triangle also forms a rectangle that has the second longest length of the Kepler scalene triangle as the longer length of this rectangle. Remember that the longer length of this rectangle with a diagonal that is also the longest length of a Kepler scalene triangle is also equal to the height and breadth of the human figure. Both of the poles of the circle’s diameter are outside of the square. The square and the circle do not share the same centre.
The hands of the human individual can also be moved to touch the circumference of the circle with limited results including the hands being outside the square when the hands touch the circumference of the circle. Only the hands can touch he circumference of the circle and not the human individual’s feet.
• Please remember again the important fact that the distance between the human individual’s outstretched arms is equal to the height of the human individual from the top of the head to the bottoms of the feet. Because the distance between the outstretched arms of a human individual is equal to the total height of a human individual from top of the head to the bottoms of the feet a square can be drawn around the physical figure of the human individual.
Another version of the creation of a circle with a circumference equal to the perimeter of a square can be made involving the height and breadth of the human individual being contained in the square with a perimeter equal to the circumference of a circle .The creation of a circle with a circumference equal to the perimeter of a square can be made involving the height and breadth of the human individual being contained in the square with a perimeter equal to the circumference of a circle when 1 of the poles of the circle’s diameter are located on the bases of the feet of the human individual. The height of the human individual divided by the radius of the circle with a circumference of equal measure to the perimeter of the square containing the height and breadth of the human individual is 1.57. 1.57 is half of Pi. The diameter of the circle divided by the height of the human individual is 1.27.
1 of the poles of the circle’s diameter touch the soles of the human individual’s feet in this version of squaring the circle with the human figure. Very important thing to remember with this version of squaring the circle with the human figure is that none of the 4 corners of the square can touch the circumference of the circle that is equal to the perimeter of the square that contains both the height and breadth of the human individual.
Both hands and feet can touch the circumference of the circle that is equal to the perimeter of the square that contains both the height and breadth of the human individual.
The height and breadth of the human individual is equal to the shortest length of a Kepler scalene triangle when the diameter of the circle that has a circumference equal to the perimeter of the square that contains both the height and breadth of the human individual is taken as the second longest length of a Kepler scalene triangle.
• The illustrator of the human figure must remember again that the distance between the tips of the longest fingers on both hands with both arms stretched out horizontally is equal to the standing height of the human individual. A square can be constructed when the height of a standing human individual is compared to the distance between the fingertips on the stretched out straight arms. Remembering that the distance between the outer edge of the shoulder trapezius muscle and the outer edge of the clavicle will help the illustrator to attach the arms to the ribcage properly and remind the illustrator that a square can be constructed from the height of the standing human individual when both arms are stretched out horizontally with longest fingertips. The distance from the tip of the longest finger on the hand to the horizontal centre of the pit of the neck when the arm is stretched out is equal to half the standing height of the human individual between the top of the head and the bases of the feet.
• Also if the height of the human figure is divided into the ratio 1.666666666666667 the longer part of the division can be used as the radius of a circle that is made of 2.4 equal units, with both the outstretched legs and arms with longest fingertips touching the circumference of a circle with limited results, including the longest fingertips of the outstretched arms aligning with the top of the head and a equilateral triangle being formed between the outstretched legs of the human individual with one fourteenth being subtracted from the stature of the human individual . 1666666666666667 is the first approximation of the Golden ratio of 1.618. This circle has a diameter of 4.8 equal units when compared to the total standing height of the human figure. Another method for achieving the radius of this circle in the correct point on the human figure is to divide the height of the human figure into equal fourths (quarters) and multiply by 2 point 4.
• Also if the height of the human individual is divided into 4 equal units of measure and a circle that has a diameter of 5 equal units of measure is constructed with 1 of its poles being in the same place as the bases of the human feet of the human individual then the longest finger tips of the human’s outstretched arms can touch this circle’s circumference that has a diameter of 5 units of equal measure, while the height f the human individual is 4 units of equal measure. Please note that if the height being the square is 4 cubits and the radius of the circle is 2 and half of 1 cubit then when 4 is divided by 2 and a half of 1 the result is 1.6 and 1.6 is the second approximation of the Golden ratio of 1.618. Another method for achieving the radius of this circle in the correct point on the human figure is to divide the height of the human figure into equal fourths (quarters) and multiply by 2 point 5.
• Please note the 2 methods mentioned above are similar and need to be distinguished by the fact that the latter method for obtaining a circle and a square for the human figure is 5 cubits for the circle’s diameter and the edge length of the square being 4 cubits and the upper 2 corners of the square must touch the circumference of the circle, while the prior method does not allow the 2 upper corners of the square to touch the circumference of the circle. Remember that the prior method has a circle with a diameter that measures 4.8 equal cubits when compared to the human’s full standing height of 4 equal cubits.
• If a circle with a radius equal to half the total height of the human figure is constructed around a human individual and is then divided into 5 equal sections a pentagon will be constructed around the human individual with the hands almost touching the arms of the pentagram and the points of the pentagram’s feet touching the insteps of the human individual’s feet and also the point of the pentagram’s main alpha touching the top of the human individual’s head.
• If the length of the arm with the hand is placed above the head and the distance between longest fingertip and the bases of the feet of the total standing human figure are divided into the Golden ratio then the distance between the bases of the feet and the centre of the chest can be the larger part of the Golden ratio.
• If 4 45 degree angles are plotted from a point around the navel located 2.4 cubits up from the base of the feet of the total height of the standing human figure until the 2 longest finger tips touch 2 corners of a square surrounding the human figure then the tips of the 2 longest toes of the feet can touch the other 2 corners of the square that surrounds the human figure with the legs and feet and calves turned inwards facing opposite directions.